Texture and colour analysis
Mathematics of textures
Texture analysis has been an area of intense research activity for more than four decades, but most of its mathematical underpinnings have been largely overlooked. In this context we are trying to define new texture descriptors based on mathematical concepts such are space partitioning, partial orders and polytopes. We are also interested in the rigorous treatment of bag-of-words models, and in particular their invariance properties under group actions such as rotations and reflections.
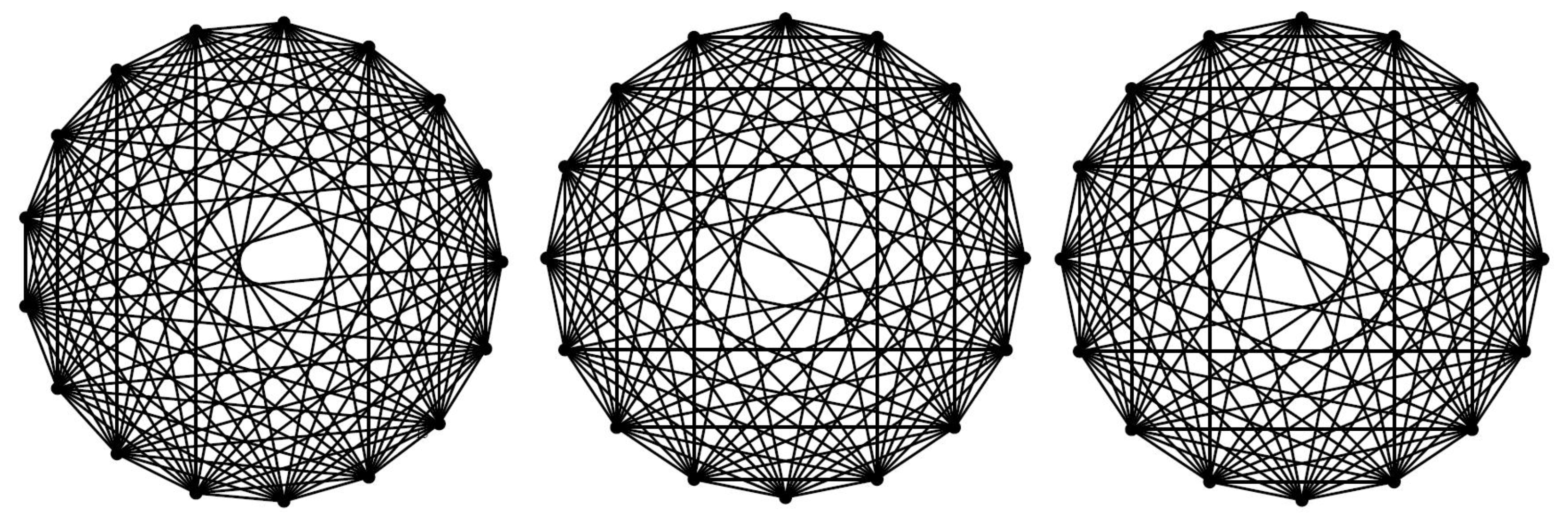
In the picture: graphs of polytopes corresponding to the following local binary patterns (LBP3x3): 00000000, 00000101 and 00001101. From Bianconi, F., Fernández, A. On the occurrence probability of local binary patterns: A theoretical study
(2011) Journal of Mathematical Imaging and Vision, 40 (3), pp. 259-268.
Colour texture descritpors
Colour has proved to improve texture analysis in many contexts. Our main interest in this area is the development of new image descriptors which combine colour and texture in effective ways. We have proposed novel colour texture descriptors such as colour ranklets, multilayer coordinated cluster representation, improved opponent-colour local binary patterns (IOCLBP) and partial-order rank features in colour space.
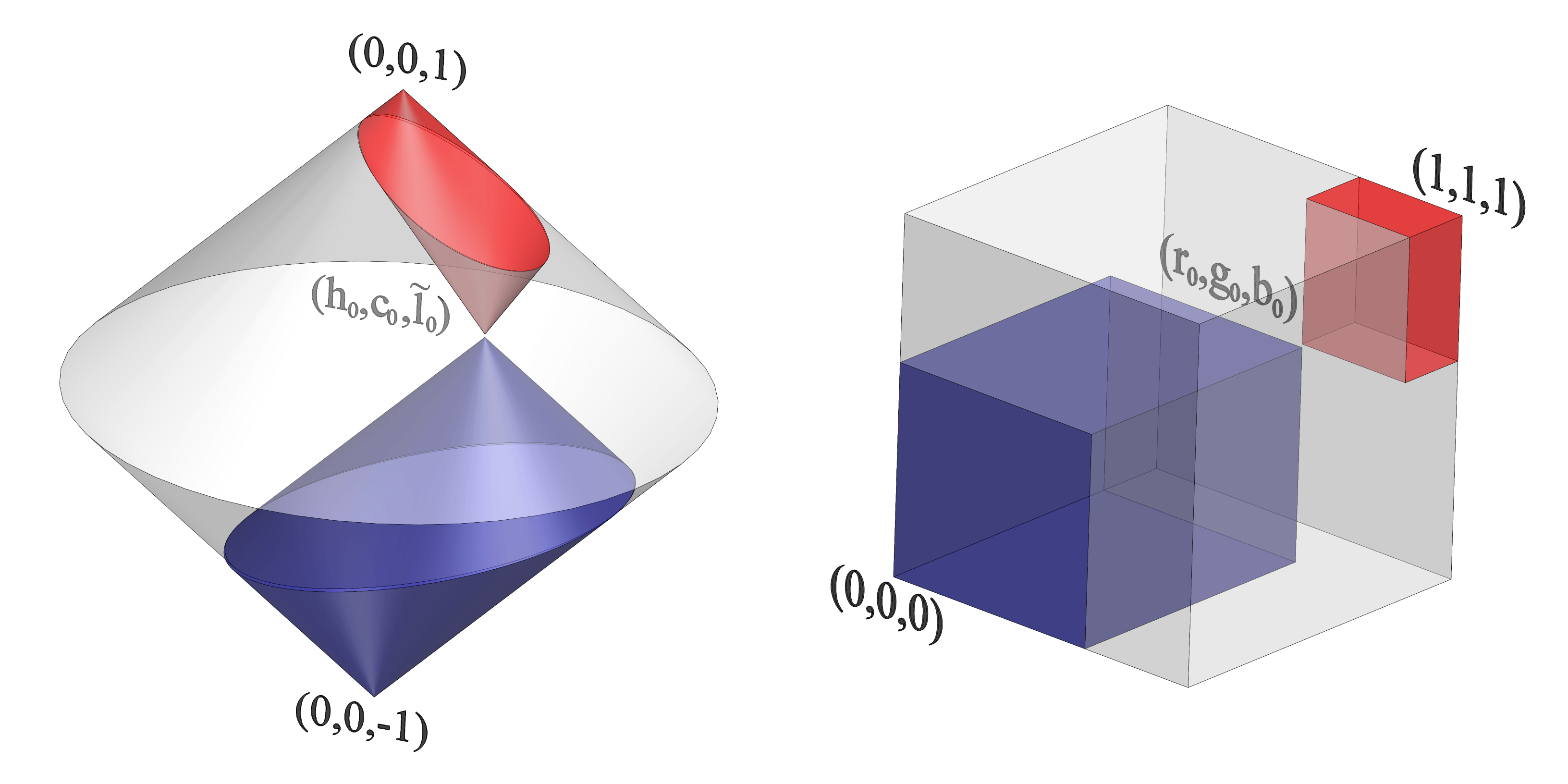
In the picture: Loewner order in the HCL space (left) and product order in the RGB space (right). From F. Smeraldi et al., Partial order rank features in colour space, Applied Sciences, 10 (2), pp. 499, 2020

